The Binomial option pricing model is a “lattice-based” or “tree shaped” model. The estimated future price of the underlying share is calculated forward until the expiry date, at distinct steps in time. As the share price could go either up or down, the results of these calculations gives the lattice or tree shape that the model is well known for.
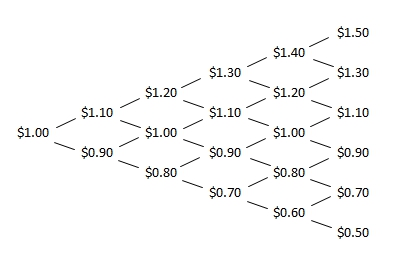
From these prices, the resulting option value is then discounted back to the current time to arrive at an estimated option value.
The Binomial option pricing model differs from the Black-Scholes model in that it cannot be worked out using a simple mathematical equation, but requires calculations for each step used in the model. This also gives it an advantage over the Black-Scholes model, in that it can also be customised for early exercise of the options by employees, as well as giving more accurate results for dividend paying shares.
Where the option has more complicated features, such as multiple variable features, the Binomial option pricing model is less practical than a model such as a Monte Carlo Simulation, however it is not as time consuming.
Contact Value Logic to find out more about which option valuation method is appropriate for you.
